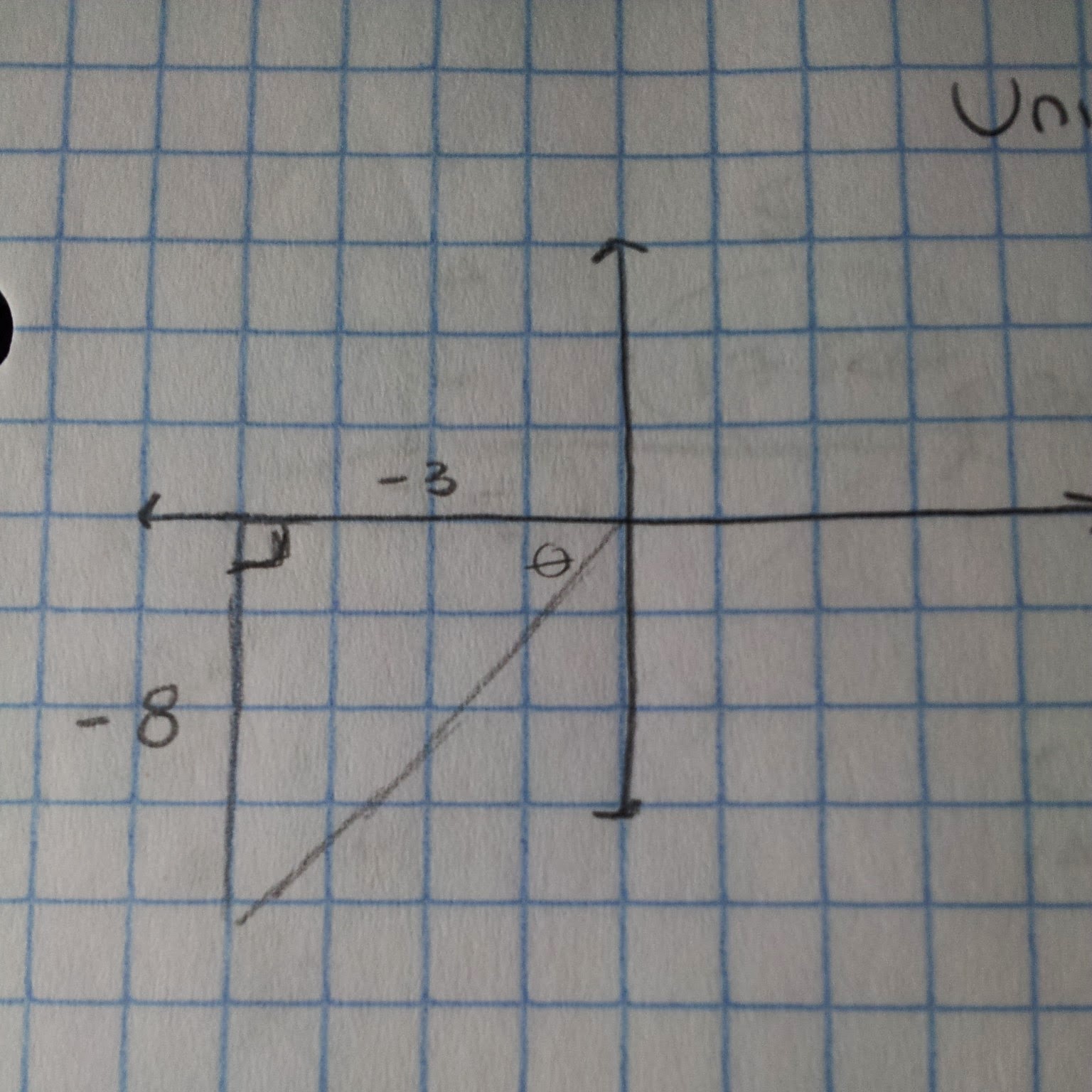SP7: Unit Q Concept 2 - Finding Trig Functions When Given One Trig Functions and Quadrant

This SP7 was made in collaboration with Molinda Av.  Please visit the other awesome posts on their blog by going here.

The Problem: tan of theta is 8/3; sin of theta is less than 1. 

So the first thing to look at is finding what quadrant the problem lies in. So tangent is positive, so that means it can be in the Quadrant 1 or Quadrant 3. Sine is less than 1 meaning that the answer will be negative, so that means it can lie in Quadrant 3 or Quadrant 4. Because they both land in Quadrant 3, then the Quadrant our answers are in lie in Quadrant 3.

We are given tangent in the problem, so we can use the reciprocal identity to find cotangent: cotangent of theta equals 1/tangent of theta. Afterwards, because we know cotangent, we can use the Pythagorean Theorem, then we can use 1+cot^2theta = csc^2theta to find cosecant.

We now know tangent (given), cotangent and cosecant, we can then use the reciprocal identity to find sine. 

We now have tangent (given), cotangent, cosecant, and sine. We have tangent as a given so we can also use the Pythagorean Theorem 1+tan^2theta = sec^2theta to find secant. 

We know tangent (given), cotangent, cosecant, sine, secant, and we need to find cosine. Because we found secant, we can then use the reciprocal identity to find cosine: cosine of theta equals 1/secant of theta. 

However, this problem can also be solved by using SOH CAH TOA. Tangent is the ratio of sine over cosine which is y/x. You can graph it out on and you would get a right triangle. The sides are negative the coordinates are negative in the third quadrant. By using the ratios, we can find the same answers as the ones when we used identities.

To solve using SOHCAHTOA, we must find the hypotenuse using the Pythagorean Theorem. 

We have found the hypotenuse so now we can use the ratios to find sine, cosine, and tangent. Sine has the ratio of y/r, cosine has the ratio of x/r, and tangent has the ratio of y/x. Remember to rationalize when you have a radical as a denominator!

To find cosecant, secant, and cotangent (which are the reciprocals of sine, cosine, and tangent), we use the ratios shows in the picture and plug in the corresponding numbers. 

I/D3: Unit Q - Pythagorean Identities

INQUIRY ACTIVITY SUMMARY:

The formula in this unit is sin^2x+cos^2x=1. Thinking back to the unit circle, the Pythagorean Theorem is x^2+y^2=r^2. However, when put into the triangle in the unit circle, x=cos and y=sin and for "r" to be 1 we have to divide everything by r^2. We are then left with (x/r)^2+(y/r)^2=1 (are those ratios looking familiar?). Th ratio for cosine is (x/r) and the ratio for sine is (y/r), so we substitute cosine and sine for x and y respectively. That is how the formula sin^2x+cosine^2x=1 is derived. 

a) Deriving the identity with Secant and Tangent. Deriving from the sin^2x+cos^2x=1. Because we are looking for secant and tangent, which is the reciprocal of cosine and the ratio for tangent is y/x (sine over cosine), I would divide everything by cosine so that 1/cosine would be secant and the sine over cosine would be tangent.

b) Deriving the identity with Cosecant and Cotangent. Deriving from the sin^2x+cos^2x=1. Because we are looking for cosecant and cotangent, I would divide everything by tangent because sin because x/y (cosine over sin) is the ratio for cotangent and 1/sin is cosecant.

INQUIRY ACTIVITY REFLECTION:

• The connections that I see between Units N, O, P, and Q so far are the Pythagorean theorem used from the Unit Circle from the Unit N and O can help us derive the formulas for Unit Q. Anther connection that is used throughout the units is the ratios, no matter what side length it is or if its a triangle not from the unit circle, the ratios remain the same throughout.
• If I had to describe trigonometry in THREE words, they would be intimidating, confusing, and rewarding. Intimidating and confusing sort of combine together because once you first start trigonometry, you feel overwhelmed by the information so you feel confused. However, I also describe it as rewarding because then you start learning various ways to remember the formulas and find out how derivation can help you if you forget certain formulas. It's rewarding in a sense that you learn different ways to learn formulas and a way to use your knowledge to derive the formula out of information you already know.

WPP #13-14: Unit P Concept 6&7 - Applications of Law of Sines and Law of Cosines

Please see my WPP #13-14 made in collaboration with Molinda Av, by visiting their blog here. Also be sure to check out the other awesome posts on their blog.

BQ#1 - Unit P - Law of Sines and Area of an Oblique Triangle

i. Law of Sines - We need the Law of Sines in order to find missing angle(s) and missing side(s) of a triangle that is not a right triangle. We can use trig functions to derive the Law of Sines.

Law of Sines: In the triangle ABC, SinA/a = SinB/b = SinC/c

When using the law of sines, we only use two out of the three.

You have a non-right triangle and draw a perpendicular line straight down the triangle from the angle B. That will be labeled "h" for height. Because we drew that perpendicular line, we now have two right triangles. We can now apply our trig skills.

To find the Law of Sines from the two triangles, we must use Sin. So using angle A, we have SinA = h/c (opposite over hypotenuse) and we have SinC = h/a (also opposite over hypotenuse). We then multiply each by the denominator to get rid of the ratio. We would then have cSinA=h and aSinC=h. Now, because both equal "h", that would also mean that they equal each other. So we set cSinA = aSinCWe and would then divide each by ac and that would cross out the a in aSinC and the c in cSinA. We would then be left with SinA/a = SinC/c.

iv. Area of an Oblique Triangle - We use this when we have SAS.

The area formula for a right triangle is A=1/2bh. The area of an oblique triangle is derived through also sin. For example, in this picture we will use angle C in the triangle that has a perpendicular line draw through it.

We have to find the "h" height. We know that sinC = h/a and that aSinC = h. So since we do not know what "h" is, we can substitute "h" for aSinC. Therefore, we would then get A=1/2baSinC. This relates to the original formula we are familiar with because it uses the same format of 1/2bh, but instead of being stuck with not know two variables (A and h), we can use our knowledge of the Law of Sines to see that h would equal aSinC and that we substitute aSinC (and the answer we have for aSinc) for "h".

WPP #12: Unit O Concept 10 - Calculating Elevation and Depression

Outing at a Ski Resort!

a) Amber is at a ski resort with her friends. As they are admiring the view of the scenery from their balcony that is 125 feet above the ground, she sees her friend arriving late. She looks down at an angle of 48*. How far is the distance from her friend and herself?

You have to use sin to find the missing side. You have sin48 = 125/x. You have to rid of the x by itself, so you multiply both sides by x (to get it to the other side and get rid of the x on the bottom) and then divide both sides by sin48 to get the x by itself. 

b) Amber goes downstairs to help her friend bring up her luggage. Their other friend, Alex, calls to them from the balcony. Amber looks up at an angle of 63*. How far is the distance between Amber and Alex?

It is the same idea as part a; you have to solve for the missing side, so you must use sin. The x is on the bottom again so you multiply both sides by x and then divide everything by sin63 to get the x. 

*Students can either use the full height of 125 feet, or can assume that the eye level of the person is 5 feet and use 120 feet as the height as well.*

I/D #2: Unit O - Deriving the SRTs

INQUIRY ACTIVITY SUMMARY

30-60-90: The equilateral triangle has all 60* angles and is labeled to have the length of 1 on all sides. You are looking for a 30-60-90 triangle so you cut the triangle in half vertically. You will have a 30* angle, a 60* angle and a 90* angle.

You see that because you had cut the triangle in half, the side across from 30* is 1/2 and the side across from 90* is 1.

You then half to find the hypotenuse, so you use the Pythagorean Theorem to solve for the missing side. 

The side across from 60* ends up being radical 3 over 2. To get rid of the fractions, you multiply everything by 2. You end up with 30* being 1, 90* being 2 and 60* being radical 3. The "n" that is used is to express that any number can be put in place of "n". Meaning that the 30-60-90 triangle can have any lengths, bigger or smaller, than the triangle used in this example and that it will still have the same pattern as long as it is a 30-60-90 triangle. Through this pattern, when there is a 30-60-90 triangle, the side across 30* will be "n", while 90* will be 2 times "n" and 60* will be "n" times radical 3.

45-45-90: The equilateral square has sides all the length of 1. To change this into a 45-45-90 triangle, you have to cut it diagonally to get the 45-45 degree angles.

You see that because you had cut the square diagonally, both the side across from 45* and 45* are still 1. So now you have to use Pythagorean Theorem to solve for the missing side, 90*.

The missing side across 90* ends up being radical 2. The "n" is used to express that there are many numbers that can be used in "n" place. The lengths of the triangle can be longer or shorter than 1 and will still maintain the same pattern as long as it is a 45-45-90 triangle. Through this pattern, when there is a 45-45-90 triangle, the sides across from both 45* and 45* angles will be "n" while the side across form 90* angle will be n times radical 2.

INQUIRY ACTIVITY REFLECTION

Something I never noticed before about special right triangles is how the pattern is determined. I faintly remember how the Pythagorean Theorem was derived, but looking at how using the Pythagorean Theorem can help derive how the pattern is created is interesting.
Being able to derive these patterns myself aids in my learning because if for some reason I forget the pattern, I can draw the triangle/square and derive the pattern out and use that instead of guessing the pattern.